The 12 Notes of Music...
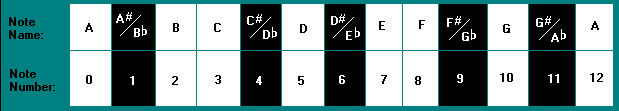
From a theoretical point of view, (western) music is essentially made up of twelve tones. These 12 tones are sometimes called the chromatic scale. We use the first seven letters of the alphabet in conjunction with some other symbols to represent these tones which are commonly called notes. As you can see from Figure 1, the pattern of notes repeats after every twelfth note. The distance between the first A (note number 0) and the second A (note number 12) is called an octave.
A real easy way to play this scale is to start on the open A string or 5th string of your guitar and work your way up the string one fret at a time. Consult the following diagram:
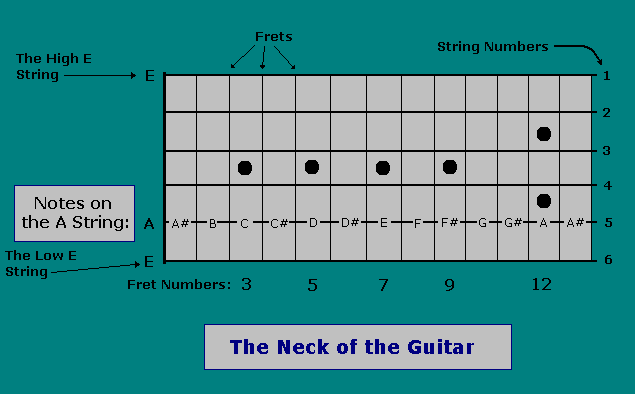
Notice that the note numbers and note names from both of the above figures have the same correspondence. (e.g. a C is associated with the number 3, a D is associated with the number 5, and so on.) I planned it to work this way to help simplify things for you.
Also be aware that the Low E string on the guitar is the string that produces the lowest sounding note (or pitch) on the guitar; it's the thickest string. It's not the string closest to the floor when you play - that's the high E string which happens to be the thinnest string on the guitar.
You'd be surprised how many times I've come across students who thought the "low-ness" of a string corresponded to the height the string was from the ground or floor. It doesn't work that way. Relative "high-ness" and "low-ness" of a string corresponds to the string's pitch.
Accidentals...
If you study Figure 1 above well enough, you'll see that seven of the twelve note names are solely comprised of alphabetic symbols, the first 7 letters of the alphabet. These notes are called natural notes. To represent the rest of the notes, symbols called sharps, ♯, and flats, ♭ are used. These symbols are called accidentals.
Sometimes we need to cancel or negate a sharp or flat - that is, turn a "sharped" or "flatted" note back into a natural note. To do this we use the natural sign, ♮. This symbol cancels out any previous occurrences of a sharp or flat for that note in a measure of music. Don't worry if the previous sentence doesn't make complete sense right now, I'll elaborate on this subject in a later lesson. For now, simply realize that seven of the twelve notes are natural notes while the others are not because they contain accidentals.
Please Note: Technically speaking, a natural sign also falls under the category of accidentals, but when most people use the word "accidental" they usually mean it to refer to a "sharp" or a "flat."
Intervals...
The distance between any two notes is called an interval. There are many different kinds of intervals, but for now, I'll just mention two:
- half-steps
- whole-steps
If we take a closer look at the chromatic scale we see that the distance between each adjacent note in the scale is the same. We call this distance a semi-tone or a half-step. NOTE: Moving a half-step on the guitar is equivalent to moving up or down one fret's distance while staying on the same string.
For example, the distance between A and A♯ is a half-step. The distance between A♯ and B is also a half-step. So is the distance between B and C, C and C♯, C♯ and D, and so on. Study Figure 1 again to be sure you understand this idea. The distance between each adjacent note in the chromatic scale is the same, a half-step.
Remember fractions from school? 1/2 + 1/2 = 2/2 = 1. Right? Works the same way with half-steps. Two half-steps equals one whole-step.
For instance, we know that the distance between C and C♯ is a half-step. We also know that the distance between C♯ and D is a half-step. So, it only makes sense then that the distance between C and D is a whole-step.
Whole-steps are equivalent to moving two frets on the same string on the guitar. For example, play the following two notes: first play the C note at the 3rd fret of the guitar on the 5th string. Now move up two frets higher on that same string. That second note is a D and it is a whole-step above C. Consult Figure 2 for assistance.
Another Thing to Beware Aware Of: Playing chromatically means to "make use of semi-tones or half-steps." This is a very loose definition, but it is more or less correct. I just want you to be aware of this term.
Chromaticism is used quite often in jazz improvisation. It basically means that one uses all 12 notes from western music to solo or improvise. And this takes a long while to get down as you can imagine. Most players only use only 5 to 7 notes when they're playing. (More on this later when I talk about other kinds of scales.)
How accidentals relate to intervals: If you haven't figured this out by now, a sharp raises a note's pitch by a half-step while a flat lowers it by a half-step. For instance, let's examine the interval between C and D♭. It's a half-step. But, if we raise the D♭ (the top note of the interval) to a D♮ the interval increases and becomes a whole-step. Now, if we take the whole-step interval and raise the bottom note, the C, to a C♯, the distance between the notes gets smaller again and becomes a half-step. Consult the following figure.
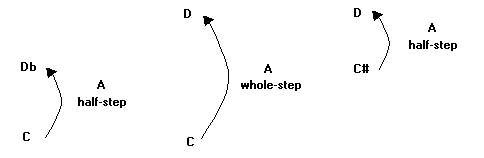
Enharmonic Spellings...
Notes that sound the same, but are written differently are called enharmonic equivalencies or enharmonic spellings. In other words, some notes can be called by different names.
This concept also applies to intervals as we will see later on.
Some note names are more appropriate than others. The name really depends on the context in which the note is played. I won't go into detail about this concept right now because it would require me to explain a considerable amount of music theory to you right now. Let me just say, however, that the concept of enharmonic spellings will become more apparent to you as your understanding of music theory deepens.
For now, I just want you to be aware that this concept exists. Also, I want you to start to become familiar with the following list of common enharmonic spellings.
Please Note: === means "is enharmonically equivalent to" or "is an enharmonic spelling for"
| A♯ | === | B♭ |
| C♯ | === | D♭ |
| D♯ | === | E♭ |
| F♯ | === | G♭ |
| G♯ | === | A♭ |
What this table of enharmonic spellings implies is that I could have drawn Figure 2 in the following way:
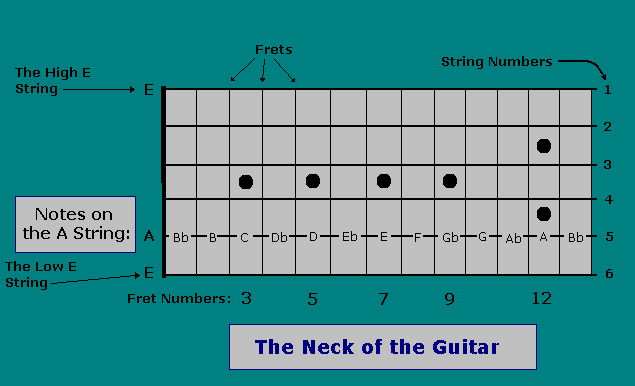
In Figure 2, I'm referring to the notes at the 1st, 4th, 6th, 9th, 11th and 13th frets using their "sharped" enharmonic spelling. In Figure 4, however, I'm using flats to refer to these notes. Both drawings mean the same thing. Simple enough, no? If this lesson makes sense, you are now ready to proceed to the next lesson.
Go to the next lesson, Lesson 2, or go back to the main menu.