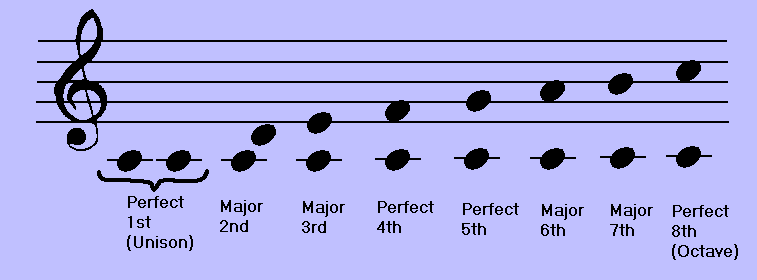
Diagram 1 - 8 Diatonic Intervals in the Key of C Major

| Interval Names | Commonly Used Symbols | Number of Half Steps | Examples Relative to C |
|---|---|---|---|
| Diminished 1st [an eharmonic spelling for a m2] |
dim. 1, °1 | -1 | C1 to Cb1 |
| Perfect Prime (Unison) | 1, P1 | 0 | C1 to C1 |
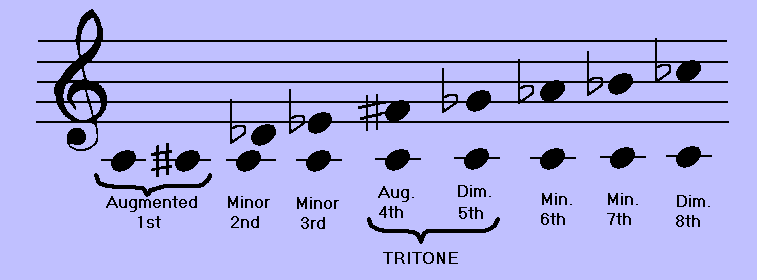
| Augmented 1st [an eharmonic spelling for a m2] |
aug. 1, +1 | 1 | C1 to C#1 |
| Diminished 2nd [an eharmonic spelling for a P1] |
dim. 2, °2 | 0 | C to Dbb |
| Minor 2nd | m2, -2, b2 | 1 | C to Db |
| Major 2nd | M2, 2 | 2 | C to D |
| Augmented 2nd [an eharmonic spelling for a m3] |
aug. 2, +2 | 3 | C to D# |
| Diminished 3rd [an eharmonic spelling for a M2] |
dim. 3, °3 | 2 | C to Ebb |
| Minor 3rd | m3, -3, b3 | 3 | C to Eb |
| Major 3rd | M3, 3 | 4 | C to E |
| Augmented 3rd [an eharmonic spelling for a P4] |
aug. 3, +3 | 5 | C to E# |
| Diminished 4th [an eharmonic spelling for a M3] |
dim. 4, °4 | 4 | C to Fb |
| Perfect 4th | P4 | 5 | C to F |
| Augmented 4th (Tritone) [an eharmonic spelling for a b5] |
aug. 4, #4, +4, TT | 6 | C to F# |
| Diminished 5th (Tritone) [an eharmonic spelling for a +4] |
dim. 5, b5, °5, TT | 6 | C to Gb |
| Perfect 5th | P5 | 7 | C to G |
| Augmented 5th [an eharmonic spelling for a m6] |
aug. 5, #5, +5 | 8 | C to G# |
| Diminished 6th [an eharmonic spelling for a P5] |
dim. 6, °6, bb6 | 7 | C to Abb |
| Minor 6th | m6, -6, b6 | 8 | C to Ab |
| Major 6th | M6, 6 | 9 | C to A |
| Augmented 6th [an eharmonic spelling for a m7] |
aug. 6, +6 | 10 | C to A# |
| Diminished 7th [an eharmonic spelling for a M6] |
dim. 7, °7, bb7 | 9 | C to Bbb |
| Minor 7th | m7, -7, b7 | 10 | C to Bb |
| Major 7th | M7, 7 | 11 | C to B |
| Augmented 7th [an eharmonic spelling for a P8] |
aug. 7, +7 | 12 | C to B# |
| Diminished 8th [an eharmonic spelling for a M7] |
dim. 8, °8 | 11 | C1 to Cb2 |
| Perfect 8th | P8 | 12 | C1 to C2 |
| Augmented 8th [an eharmonic spelling for a m9] |
aug. 8, +8 | 13 | C1 to C#2 |