Lesson 6: The Major Scale (Part 4) and the Circle of 5ths
Lesson Contents:
- How To Construct Any Major Scale
- Key Signatures
- The Circle of 5ths
- The Notes in the 15 Major Keys
Introduction
In this lesson I would like to elaborate on some points that were made in Lesson 5
and in some earlier lessons. I am going to talk about more intervalic concepts by revisiting
the major scale. This lesson won't be as long as the previous one. Sorry about that, but
it is necessary to look at music from a very low level before we can approach higher
level concepts. There are a lot of little details that need to be examined. This lesson
will still look at music from a pretty low level, but I will also start to present some
higher level concepts that are essential for understanding improvisation.
How To Construct Any Major Scale
In previous lessons I have presented a couple of different ways how to
play any major scale, but now I would like to show you how to
construct any major scale using a theoretical approach. This
will enable you to figure out which enharmonic spellings to use for any major scale. This
may not seem important, but it actually is because it forms the basis for understanding
key signatures and something called the
Circle of 5ths - two things I will explain later in
this lesson. The following method, for instance, will explain why we call the third note
of an E major scale a G# instead of an Ab.
The best way to explain this method is by working with an example. Let's construct
the E major scale:
-
First, write out 8 consecutive notes without any accidentals starting
with E. Please note that the ending note of the scale will
be the same as the starting note except it will be one octave higher. In the case of
E major, our starting note is E and by
adding 7 consecutive natural notes we get:
E, F, G, A, B, C, D,
and E.
-
Now using the major scale formula as a guide (W W H W W W H)
along with the chromatic scale below in Diagram 1, apply any needed accidentals (sharps or flats)
to the 8 natural notes until we get an E major scale.

Diagram 1 - The Chromatic Scale
-
The first 2 notes: We start by comparing the first two
notes of our "natural note" scale against the first interval of the major scale formula - the
whole step. We then work our way through all 8 notes of the scale comparing consecutive pairs
of notes until we reach the last note. At that point, if we have made our calculations
correctly, that is, if we have added the correct accidentals to certain notes (some might not
change) we should then have an E major scale - our transformed notes will comply to the
major scale formula. So here we go:
The distance between E and F is a half step but we need a whole step so we raise F a half step
by sharping it to make F#. The first two notes of an E major scale are
E and F# and our 8 notes now look like this:
E, F#, G, A, B, C, D, E.
-
The 3rd note: Now let's examine the distance between F#
and G - it is another half step too, but according to the major scale formula we need
a whole step between them, so we raise G a half step by sharping it. This gives us the
first 3 notes of an E major scale: E, F#, G#.
Notice that we have maintained the integrity of the scale's
alphabet. That is why we don't call the F# a Gb or the G# an Ab. This is a key
concept and it is the rationale behind writing out the 8 natural notes in step 1. You see,
each note of a major scale must have a different alphabetic name,
but the alphabet's integrity must be maintained between adjacent notes. That is,
"some kind of E" must be followed by "some kind of F" which must be followed by "some kind
of G," etc. We can't have an E followed by a Gb, for instance.
Our 8 notes at this point now look like this:
E, F#, G#, A, B, C, D, E.
-
The 4th note: Now we need a half step between the
3rd and 4th degree of a major scale and we have a G# and an A. Hey, we're in luck.
This already is a half step and we don't need to add any accidentals to the A note.
The first 4 notes of an E major scale are E, F#, G#, and A.
And the 8 notes look like this:
E, F#, G#, A, B, C, D, E.
-
The 5th note: Now we need a whole step between the 4th
and 5th note of the scale. We have an A and a B and again we don't have to add any
accidentals because the distance between A and B is a whole step. If this is
confusing be sure to consult Diagram 1 to aid you when counting half steps between notes or
read my previous lessons if you haven't already. At this point we have the first 5 notes
of an E major scale: E, F#, G#, A, and B. And the 8 notes of
our almost transformed scale look like this:
E, F#, G#, A, B, C, D, E.
-
The 6th note: We need a whole step. We have B and C.
This is a half step, so we sharp C. We get: E, F#, G#, A, B and C#.
And the 8 notes look like this:
E, F#, G#, A, B, C#, D, E.
-
The 7th note: We need another whole step. We have C# and D.
This is a half step, so we sharp D. We get: E, F#, G#, A, B, C# and D#.
And the 8 notes look like this:
E, F#, G#, A, B, C#, D#, E.
-
The 8th note: We need a half step. We have D# and E.
This is a half step, so we don't have to do anything. We get: E, F#, G#,
A, B C#, D# and E. We know we are correct because we ended with an E note and
this indeed is the correct ending note for an E major scale.
The final scale looks like this:
E, F#, G#, A, B, C#, D#, E.
Key Signatures and the Circle of 5ths
From previous lessons we know that a C major scale has no sharps or flats. It follows then
that the key of C major has no sharps or flats in its key signature because the key of C major
is comprised of notes from the C major scale. A scale and a key are
basically the same thing!
A key signature tells
us which and how many accidentals a key (or scale) has. For instance,
in the case of E major we have 4 accidentals, F#, C#, G#, and D#. And it is these
4 accidentals that are used to identify this scale or key. No other major scale
has this kind of key signature. In short, each major scale has
a unique key signature which is used to distinguish it from all the other major scales
and keys.
The "key" point... A key is simply a scale
that creates a basis (or ingredients) for a tonal center for a piece of music.
As you will discover again and again, scales and chords are all basically the same thing - they
are just 2 different ways in which we can express the same notes. Scales being melody - single
notes played one at a time, and chords being harmony - notes stacked
on top of each other played at the same time. Keys, chords, scales - they are all the same thing
from a certain point of view.
If we employ the above method for deriving major scales starting with any note from the chromatic
scale, some patterns will start to emerge. These patterns are organized in the following chart called the
Circle of 5ths.
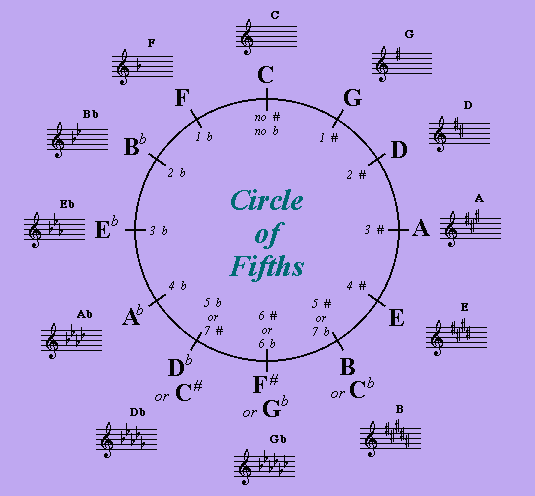
Diagram 2 - The Circle of 5ths
How to interpret the circle of 5ths... First, start at the top note (key)
of the circle, the C. This part of the diagram tells us that the key of C major has no sharps or
flats in its key signature. The little picture of the staff shows a drawing of the key signature for
C major. Each note name in the circle of 5ths represents a major key name or the name of a major
scale. They are synonymous with each other. (I guess I really want to drill this point home, eh?)
Moving clockwise on the circle we arrive at the key of G major. G major is up a perfect 5th from C.
(G is the 5th note of a C major scale.) G major has one accidental in its key signature, an F#. Keep
moving clockwise and we arrive at the key of D major which is also up a perfect 5th from G. (D is the
5th note of a G major scale.) D major has 2 accidentals in its key signature, an F# and a C#. We
start to see a pattern (hopefully.) This pattern of accidentals emerging one
at a time and then disappearing one at a time continues as we go around the circle until we arrive
at the beginning again with the key of C major. (C is the 5th note of an F major scale).
You will notice that 3 locations on the circle have 2 different names. B is the same as Cb,
F# is the same as Gb and C# is the same as Db. These keys are known as enharmonic keys (keys
that sound the same, but are spelled differently). As far as practical use, you would "use"
the key of B over the key of Cb. It is easier to think of or keep track of 5 sharps rather
than 7 flats. Also you would "use" the key of Db instead of the key of C#, because it is
easier to think of 5 flats instead of 7 sharps. Since the keys of F# and Gb have an equal
number of sharps and flats, either one is game and both are used. I guess it depends on
which kind of accidental you prefer. (As my friend Rachel once said, "It's better to see
sharp than to be flat.")
Some interesting observations... The keys of C# and Cb are
considered more "theoretical" keys than actual usable keys, so for most situations you
would probably not use them. An interesting observation can be made about these two
theoretical keys, however, when we compare them to the key of C major. First, we know that
the key of C major has no sharps or flats in its key signature. It only makes sense then
that the key of C# would have all of its notes raised by one half step compared to the C major
scale. It also makes sense that the key of Cb would have all of its notes lowered by a half step
compared to the C major scale. The point I'm trying to make here is that when
you compare keys with lots of accidentals to keys without lots of accidents some insights can be attained.
For instance, the key of D major has 2 sharps in its key signature, F# and C#. Now, the key
of D flat has all of its notes flat except F and C. Makes sense. Another example: the key
of G major has only 1 sharp in its key signature, an F#. The key of G flat has all of its notes
flat except the F note. Hopefully these kinds of observations will strike you as being "kind
of cool." This kind of thinking certainly helps me to remember some of the weirder keys - the
flat keys.
Memorization Trick... Here's a little thing that can help you remember the
order in which accidentals appear in the circle of 5ths. For sharp keys remember the sentence:
Fat Cats Get
Drunk At Ed's
Bar. The first letter of each word in this sentence represents the note
that gets sharped. For instance, in the key of E we have 4 accidentals. So we think, "Fat cats get
drunk" -- F#, C#, G#, and D#.
For the flat keys just read the "fat cat" sentence backwards, or remember the
word BEAD and then say, G, C, F. Or you can use the phrase:
Big Ed And Donna Got Caught... Flirting.
Where you thinking of a different F-word? (Shame.)
The Notes in the 15 Major Keys
And so ends this lesson, however, one more thing is worth mentioning. For all major
scales, sharps and flats do not mix. That is, there are sharp keys
and flat keys, but there is no major key that contains both sharps and flats in its
key signature.
Consult the following table:
|
Key |
The Major Scales
(Note Names and Their Function) |
|
R |
2 |
3 |
4 |
5 |
6 |
7 |
R |
| The Natural Key: |
C |
C |
D |
E |
F |
G |
A |
B |
C |
| Sharp Keys: |
| G |
G |
A |
B |
C |
D |
E |
F# |
G |
| D |
D |
E |
F# |
G |
A |
B |
C# |
D |
| A |
A |
B |
C# |
D |
E |
F# |
G# |
A |
| E |
E |
F# |
G# |
A |
B |
C# |
D# |
E |
| B |
B |
C# |
D# |
E |
F# |
G# |
A# |
B |
| F# |
F# |
G# |
A# |
B |
C# |
D# |
E# |
F# |
| C# |
C# |
D# |
E# |
F# |
G# |
A# |
B# |
C# |
|
Key |
The Major Scales
(Note Names and Their Function) |
|
R |
2 |
3 |
4 |
5 |
6 |
7 |
R |
| Flat Keys: |
| F |
F |
G |
A |
Bb |
C |
D |
E |
F |
| Bb |
Bb |
C |
D |
Eb |
F |
G |
A |
Bb |
| Eb |
Eb |
F |
G |
Ab |
Bb |
C |
D |
Eb |
| Ab |
Ab |
Bb |
C |
Db |
Eb |
F |
G |
Ab |
| Db |
Db |
Eb |
F |
Gb |
Ab |
Bb |
C |
Db |
| Gb |
Gb |
Ab |
Bb |
Cb |
Db |
Eb |
F |
Gb |
| Cb |
Cb |
Db |
Eb |
Fb |
Gb |
Ab |
Bb |
Cb |
Proceed to Lesson 7 or go back to the
main menu.


