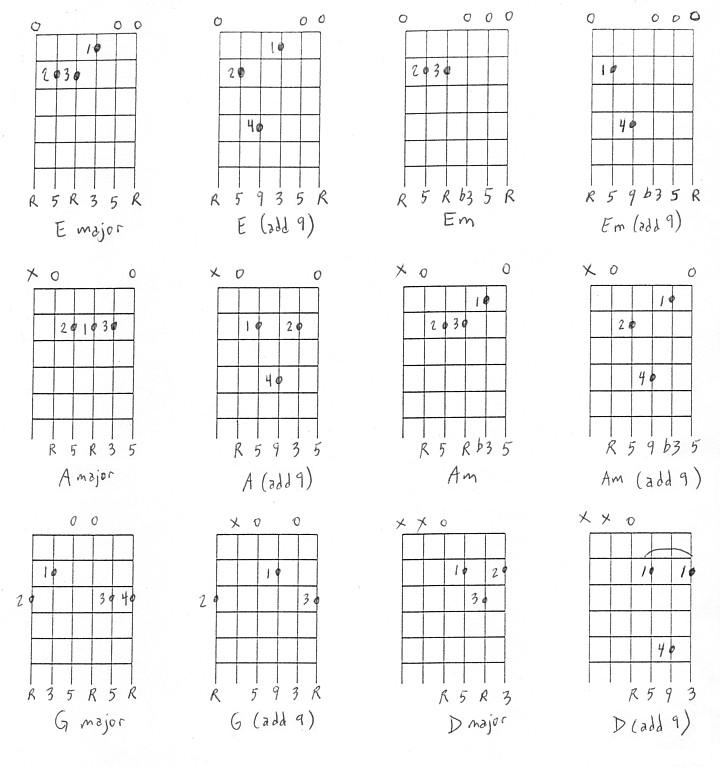
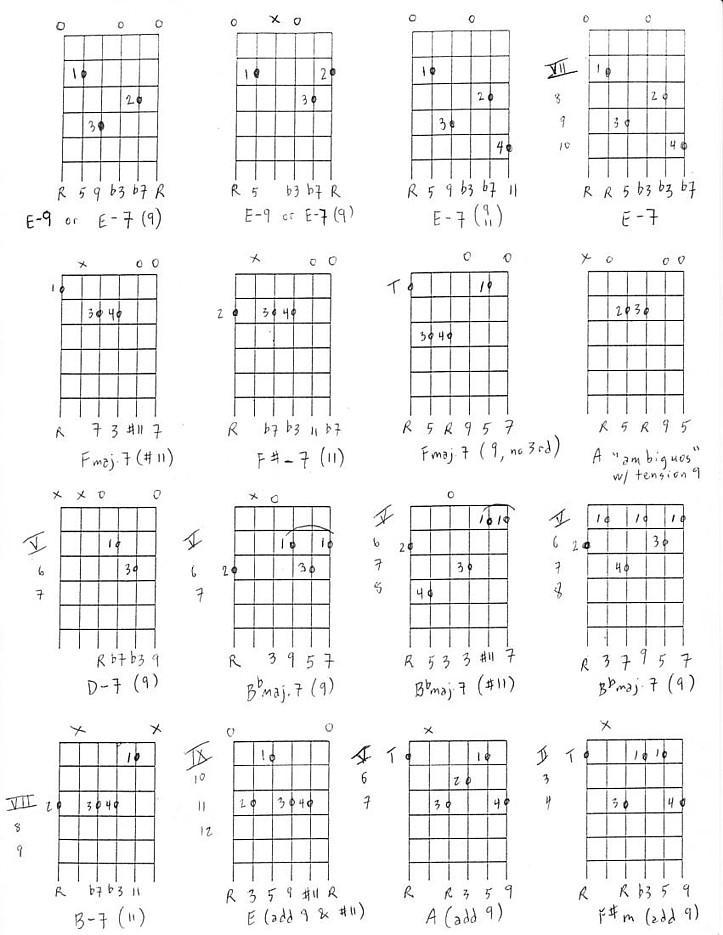
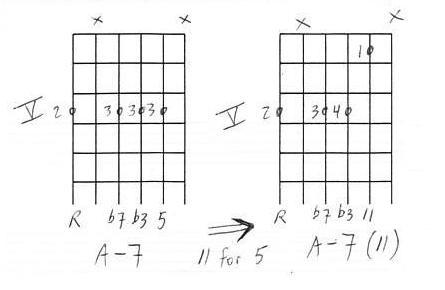
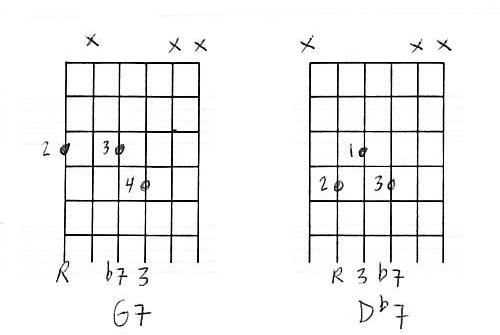
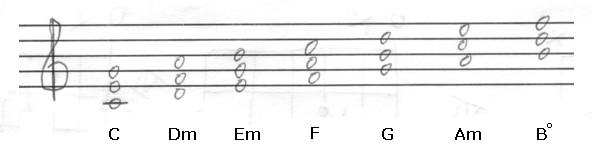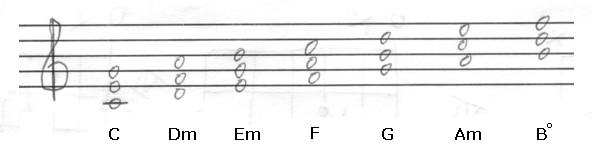|
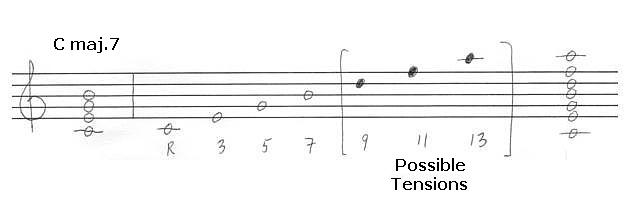
C maj.7 --> c = 1, e = 3, g = 5, b = 7,
d = 9, a = 13
[ Tension #11, an f#, is not available here because it's
non-diatonic to the key of C major. ]
D min.7 --> d = 1, f = b3, a = 5, c = b7,
e = 9, g = 11
[ Tension 13 is only available in a Dorian mode context and that should
make sense since a 13 is the same as a "raised 6th" or the characteristic note for Dorian. ]
E min.7 --> e = 1, g = b3, b = 5, d = b7,
a = 11
F maj.7 --> f = 1, a = 3, c = 5, e = 7,
g = 9, b = #11,
d = 13
G7 --> g = 1, b = 3, d = 5, f = b7,
a = 9, e = 13
[ Pretty much anything tension works over a "five" chord,
so check out the exceptions listed above and experiment with what sounds
"good" to you. Also, if you listen to a lot of Jazz music, you'll
develop a taste, or rather, an ear, for tensions and more complex harmonies
than your standard pop harmonies. ]
A min.7 --> a = 1, c = b3, e = 5, g = b7,
b = 9, d = 11
B min.7 (b5) --> b = 1, d = b3, f = b5, a = b7,
e = 11, g = b13
|